This is a sponsored post from Gatorade Sports Science Institute (GSSI). If you’re interested in partnering with ASN, learn more about the Sustaining Partner program here and advertising opportunities here.
Resting metabolic rate (RMR) is the largest contributor to total energy expenditure in all but the most physically active individuals (Mahan et al., 2012). Therefore, measuring or precisely estimating an individual’s RMR substantially supports adequate nutrition prescription of energy and macronutrient requirements.
RMR is commonly measured via indirect calorimetry (IC); however, IC is not feasible for many nutrition professionals working with patients and/or athletes due to the conditions required for ensuring accurate measurement and the costly equipment needed. Thus, prediction equations are routinely used to estimate RMR in a variety of populations. These equations are modeled from databases of measured RMR, identifying independent variables such as body mass (BM), height, age, sex, fat mass (FM), and fat-free mass (FFM).
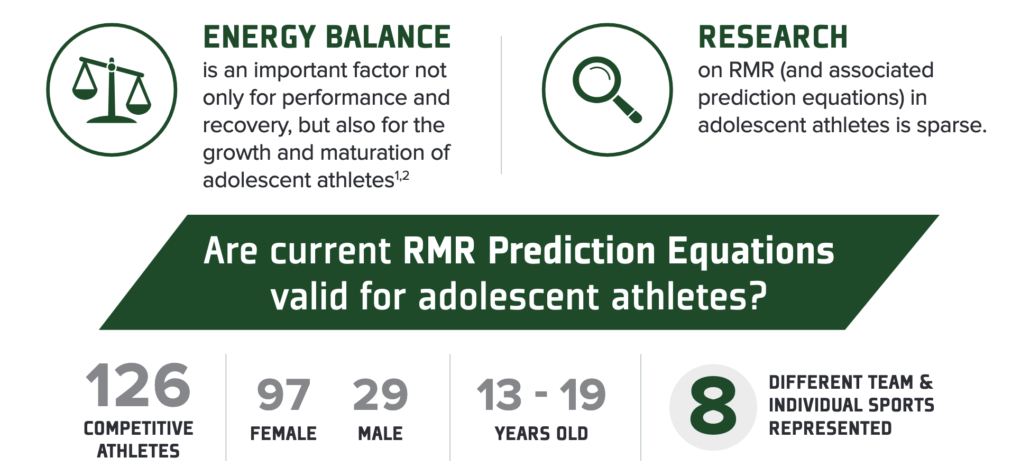
Equations exist for a variety of populations across the age span in both clinical and nonclinical populations (Food and Agriculture Organization, 2004), including athletes (Jagim et al., 2018; Schofield et al., 2019) and obese adolescents (Lazzer et al., 2006); however, research on RMR (and associated prediction equations) in adolescent athletes is sparse. Understanding energy needs, RMR specifically, of adolescent athletes is important, as they differ from nonathletes (De Lorenzo et al., 1999) and from adults (Food and Agriculture Organization, 2004).
This was the first study to assess RMR and to develop and validate prediction equation models in a large, diverse cohort of adolescent athletes with similar racial makeup to the wider United States. The following were the main findings: (a) most existing equations underestimate RMR in adolescent athletes, (b) a one- and two-compartment model developed for this study both performed better than previously published models; however, (c) there was no clear advantage in using a two-compartment model (with FFM and FM) vs. a one-compartment model (BM only) to predict RMR in this cohort.
Given these findings, the following equation would be the most suitable for predicting RMR in adolescent athletes, thereby aiding the development of sufficient nutrition prescriptions.
Males: RMR = 11.1 × BM (kg) + 8.4 × height (cm) – 340
Females: RMR = 11.1 × BM (kg) + 8.4 × height (cm) – 537
Future research should seek to assess RMR in female adolescent athletes and non-White/European American populations as this study did not adequately cover these populations.
References
De Lorenzo, A., Bertini, I., Candeloro, N., Piccinelli, R., Innocente, I., & Brancati, A. (1999). A new predictive equation to calculate resting metabolic rate in athletes. Journal of Sports Medicine and Physical Fitness, 39(3), 213–219. PubMed ID: 10573663
Food and Agriculture Organization. (2004). Human energy requirements: Report of a joint FAO/WHO/UNU expert consultation. Rome, Italy: World Health Organization, October 17–24, 2001.
Jagim, A.R., Camic, C.L., Kisiolek, J., Luedke, J., Erickson, J., Jones, M.T., & Oliver, J.M. (2018). Accuracy of resting metabolic rate prediction equations in athletes. Journal of Strength and Conditioning Research, 32(7), 1875–1881. PubMed ID: 28682934 doi:10. 1519/JSC.0000000000002111
Lazzer, S., Agosti, F., De Col, A., & Sartorio, A. (2006). Development and cross-validation of prediction equations for estimating resting energy expenditure in severely obese Caucasian children and adolescents. British Journal of Nutrition, 96(5), 973–979. PubMed ID: 17092390 doi:10.1017/BJN20061941
Mahan, L.K., Escott-Stump, S., Raymond, J.L., & Krause, M.V. (2012). Krause’s food and the nutrition care process. St. Louis, MO: Elsevier Health Sciences.
Schofield, K.L., Thorpe, H., & Sims, S.T. (2019). Resting metabolic rate prediction equations and the validity to assess energy deficiency in the athlete population. Experimental Physiology, 104(4), 469–475. PubMed ID: 30758869 doi:10.1113/EP087512




